Description
The transverse Mercator projection, also known as the Gauss-Krüger projection, is similar to Mercator except that the cylinder touches the sphere or ellipsoid along a meridian instead of the equator. The result is a conformal projection that does not maintain true directions. The central meridian is placed in the center of the region of interest. This centering minimizes distortion of all properties in that region. This projection is best suited for north-south oriented areas. The Universal Transverse Mercator (UTM) coordinate system and Gauss-Krüger coordinate systems are based on the transverse Mercator projection and the State Plane Coordinate System uses it for all north-south zones. Various countries use this projection for their topographic maps and large-scale coordinate systems.
The spherical version of the projection was presented by Johann H. Lambert in 1772. First formulas with ellipsoidal correction were developed by Carl F. Gauss in 1822. The Gauss-Krüger name refers to the ellipsoidal form reevaluated by Louis Krüger in 1912. It is available in ArcGIS Pro 1.0 and later and in ArcGIS Desktop 8.0 and later.
Projection properties
The subsections below describe the transverse Mercator projection properties.
Graticule
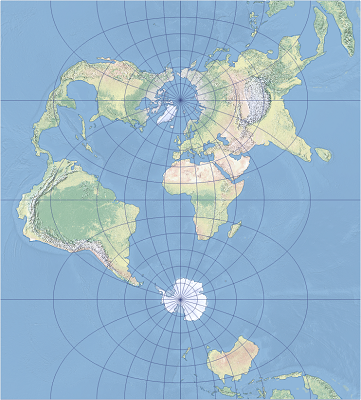
Transverse Mercator is a transverse cylindric projection. The central meridian and antimeridian lines are presented as one vertical line in the middle of the projection. Northern parts of meridians 90° away the central meridian project as a horizontal straight line through the North Pole, extending to infinity when approaching the equator. Similarly, southern parts of those meridians are projected as a horizontal line though the South Pole, also extending to infinity.
The equator is split into two parts. The first part, containing meridians within 90° of the central meridian projects as horizontal straight line in the middle of the map, splitting the projected area into two (north and south) hemispheres. The second part of the equator is projected as two straight lines at the top and bottom of the map. Similarly, all three lines extend to infinity on both ends when approaching meridians 90° away the central meridian. The other parallels are complex curves.
Both poles are projected as points, each being in the center of its hemisphere. Two points on the equator, exactly 90° away the central meridian, project to infinity and cannot be shown on the map.
Distortion
Transverse Mercator is a conformal map projection. It generally does not maintain true directions, but angles and shapes are maintained at infinitesimal scale. Distances are accurate along the central meridian if the scale factor is 1.0. If it is less than 1.0, there are two approximately straight lines with accurate scale equidistant from and on each side of the central meridian. Area, distance, and scale distortions rapidly grow with distance from the central meridian or the two standard lines as specified above. Deformations are enormous at the left and right map edges. Distortion values are symmetric across the equator and the central meridian.
Usage
The transverse Mercator projection is appropriate for mapping large-scale or smaller areas with predominantly north-south trending extents. It is a very commonly used projection. Various countries use it for their topographic maps and large-scale coordinate systems. The Universal Transverse Mercator (UTM), State Plane, and Gauss-Krüger coordinate systems all use this map projection.
Variants
There are three variants available in ArcGIS:
- Transverse Mercator is based on equations published by John P. Snyder and the Defense Mapping Agency technical manual. It is available in ArcGIS Pro 1.0 and later and in ArcGIS Desktop 8.0 and later.
- Transverse Mercator complex is based on John P. Snyder publications and a technical report by Jeff Dozier published by the National Oceanic and Atmospheric Administration. It is available in ArcGIS Pro 1.0 and later and in ArcGIS Desktop 9.0 and later.
- Transverse Mercator NGA 2014 is based on a standardization document by the National Geospatial-Intelligence Agency, revising a Defense Mapping Agency technical manual. It is available in ArcGIS Pro 1.2 and later and in ArcGIS Desktop 10.4 and later.
Limitations
Two points on the equator exactly 90° away the central meridian cannot be projected on the Transverse Mercator NGA 2014 variant. Transverse Mercator and Transverse Mercator complex variants are limited to project data only within 45° and 80° respectively from the central meridian and do not display the antimeridian due to instability in the implementations' equations. Transverse Mercator NGA 2014 projects a much larger portion of the globe, including areas along the antimeridian, but it is also limited at the left and right edges of the map.
Parameters
Transverse Mercator parameters are as follows:
- False Easting
- False Northing
- Central Meridian
- Scale Factor
- Latitude Of Origin
Transverse Mercator complex parameters are as follows:
- False Easting
- False Northing
- Central Meridian
- Scale Factor
- Latitude Of Origin
Transverse Mercator NGA 2014 parameters are as follows:
- False Easting
- False Northing
- Central Meridian
- Scale Factor
- Latitude Of Origin
Universal Transverse Mercator Coordinate System
The Universal Transverse Mercator (UTM) coordinate system is a specialized application of the transverse Mercator projection. The world is divided into 60 north and south zones, each six degrees wide. Each zone has its own central meridian and a scale factor of 0.9996. Zones 1N and 1S start at 180° west. The limits of each zone are 84° north and 80° south, with the division between north and south zones occurring at the equator.
The origin for each zone is the intersection of its central meridian and the equator. To eliminate negative coordinates, the coordinate system alters the coordinate values at the origin of the south zones. The value given to the central meridian is the false easting, and the value assigned to the equator is the false northing. A false easting of 500,000 meters is applied. A north zone has a false northing of zero, while a south zone has a false northing of 10,000,000 meters.
State Plane Coordinate Systems
The State Plane Coordinate System is not a projection. It is a coordinate system designed for the large-scale mapping in the United States. Three conformal projections were chosen for that purpose. Among them, the transverse Mercator is used for zones that are longer north-south.
Gauss-Krüger Coordinate Systems
The Gauss-Krüger coordinate system is similar to the UTM coordinate system and is used in Europe, Russia, and China. Both 3- and 6-degree-wide zones exist. Some parameter values, like the scale factor and sometimes the false easting, differ from UTM.
Sources
Dozier, J. (1980). Improved Algorithm for Calculation of UTM and Geodetic Coordinates. NOAA Technical Report NESS 81. Washington, DC: National Oceanic and Atmospheric Administration.
Snyder, J. P. (1987). Map Projections: A Working Manual. U.S. Geological Survey Professional Paper 1395. Washington, DC: United States Government Printing Office.
Snyder, J. P. and Voxland, P. M. (1989). An Album of Map Projections. U.S. Geological Survey Professional Paper 1453. Washington, DC: United States Government Printing Office.
The Universal Grids and the Transverse Mercator and Polar Stereographic Map Projections. National Geospatial-Intelligence Agency Standardization Document. Version 2.0. Available online: https://earth-info.nga.mil/GandG/publications/NGA_SIG_0012_2_0_0_UTMUPS/NGA.SIG.0012_2.0.0_UTMUPS.pdf [accessed on 10 October 2018].
The Universal Grids: Universal Transverse Mercator (UTM) and universal Polar Stereographic (UPS). Defense Mapping Agency Technical Manual 8358.2 (1989). Available online: https://earth-info.nga.mil/GandG/publications/tm8358.2/TM8358_2.pdf [accessed on 10 October 2018].